The V-slope method predicted metabolic acidosis in 10/14 who had a fall in HCO3- more than 2.5 mEq/L. A significant proportion of patients with COPD seem to develop metabolic acidosis during exercise. These coordinates may now be used to set up the following proportion in terms of the slope of the line. 1584 ft-1056 ft 2112 ft-268 ft 40 mi/hr-30 mi/hr = 50 mi/hr-V mi/hr Solving this expression for V, 528 1844 10 50-V V = 15.1 mi/hr The more visual these methods can be made, the easier it will be for the court to understand them.


Many translated example sentences containing 'v-slope method' – Spanish-English dictionary and search engine for Spanish translations. The V slope method of detection had consistently good agreement among reviewers and was least affected by the protocol. The variance in the ATge (excluding intersubject and error variance) accounted for by differences in protocol, method, and reviewer was 82%, 14%, and 4%, respectively.
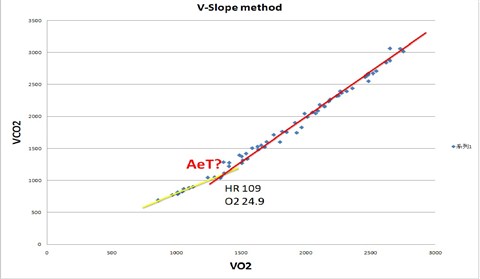
V Slope Method Definition
Algebra -> Linear-equations-> Lesson Point Slope form vs. Slope Intercept form Log On
This Lesson (Point Slope form vs. Slope Intercept form) was created by by tutoringisfun(17) : View Source, Show About tutoringisfun: I tutor Algebra 1, Algebra 2, and College Algebra. If you need more in-depth help, please visit my website listed above, or send me an email.Thanks!Point Slope form vs. Slope Intercept form
This lesson will aid students in understanding how to convert from Point Slope form to the more familiar Slope Intercept form.
A linear equation is not always written in the slope intercept form, sometimes it is not even given. Sometimes there is only a point and the slope given. In these instances,
it is necessary to use the point slope formula to obtain the slope intercept formula, so one can solve the equation.
These two formulas are shown below:
y = mx + B - Slope intercept form
(y - Y1) = m(x - X1) - Point Slope form
Example 1Write the slope intercept form for the following equation using the given slope and point: slope = 12, point ( 3,1).
Here are the steps to get the equation for this line:
1. write down the correct formula
(y-Y1) = m(x-X1)
2. substitute in the values for X1=3, m=12 and Y1=1
(y-1) = 12(x-3)
3. distribute the 12 and get rid of the parenthesis
y-1 = 12x-36
4. solve for y by adding +1 to each side making the 1's on the left cancel out
y-1+1 = 12x-36+1
5. combine like terms and watch your signs.
y = 12x-35.
This is the equation of the line in slope intercept form.
Example 2Sometimes you might need to determine if two lines are parallel or perpendicular. You can use the point slope formula to figure that out as well.
Write the slope intercept form of the line equation for the line that passes through the point (3,4) and is perpendicular to y = 3x+2.
1. write the point slope formula
(y-Y1) = m(x-X1)
2. substitute in the values from the problem for m, X1=3, Y1=4
(y-4) = -1/3(x-3)
(Remember that perpendicular lines have slopes that are reciprocals and have opposite signs of each other. So, we use the slope m = -1/3 here)
3. distribute the 1/3 to get rid of the parenthesis
y-4 = -1/3x + (1/3)*3)
4. simplyfy the right side by multiplying 3(1/3). The 3's cancel out leaving
y-4 = 1/3x+1
5. solve for y by adding 4 to each side and making the 4's on the left side cancel out.
y-4+4 = -1/3x+1+4.
This gives
y = -1/3x+5
We can check to see if these lines are perpendicular. Indeed, 1/3 is the reciprocal for 3 and -1/3 has the opposite sign, so the lines are perpendicular.
Example 3Find the equation of the line that is parallel to y = - 6x-2 and goes through the point (1,4).
1. write the point slope formula
(y-Y1 ) = m(x-X1)
2. determine the slope of the parallel line. Parallel lines have the same slope, therefore the slope of the new line is -6
3. substitute the values above for m, Y1=4, X1=1 into the formula
(y-4) = -6(x-1)
4. distribute the 6 and get rid of the parenthesis, watch your signs.
y-4 = -6x+1
5. to solve for y, we add 4 to each side and cancel out the 4's on the left
y-4+4 = -6x+1-4
6. combine like terms
y = -6x-3
7. Look at the slope of the new line to determine if it is the same as the given line
-6 = -6, therefore, the lines are parallel.
I hope this lesson has been useful. If you need more paid help you can contact me through the websites listed on my profile and I will be happy to assist you. Have a great day!
This lesson has been accessed 6378 times. |
|
V Slope Method
V-slope-method Ventilatory Threshold
Play Slope unblocked game
| SOLITAIRE ★ TIC TAC TOE ★ SNAKE★MINESWEEPER ★ AMONG US
'> |
|
The Slope is the most exciting speed game. At first glance, the game may seem simple, but you should try to play it at least once. You will not notice how you spend several hours enthusiastically playing it. The game developers have thought through every detail so that you not only play the game but also develop your reaction. The simple design of the Slope game unblocked will not overload your visual channel, so you can play it for several hours and not feel exhausted. This game is suitable for both young children and adults - it is universal and will interest any player.
The basics of the game are that you need to roll the ball as far as possible. With each passing second, the ball moves faster and faster; it makes the game so exciting and hard to play. The Slope is also complicated by the fact that there are red obstacles on the way, which break the ball and end the round. You need to learn to play in such a way that at high speed not to touch the obstacles and still allow the ball to stay on its way.
|
|
|
Why is the Slope unblocked full screen one of the best games of its kind? With each passing round, the excitement grows inside you, and it makes you want to pass the level further and further. In regular speed games, players complete a level several times in a row and stop playing at all. But this is not about our game! Even playing it a few dozen times in a row, you will still have the desire to become better and better. Another big plus of the Slope y8 unblocked new method is that you can log in to the game under your own nickname and compete on the number of points with other players. The more you play the more likely to see your name in the list of the best players. And the last but not the least advantage of the game: it is as realistic as possible. When developing the game, the laws of physics were taken into account. Therefore, playing this game feels as if you are playing a ball in real life.
Sounds interesting? Try it yourself!
|
|


